Welcome to the realm of circles! Embark on an enlightening journey with common core geometry unit 9 circle geometry answers, a comprehensive guide that unlocks the secrets of this fascinating shape. Delve into the intricacies of circles, unravel their properties, and conquer the challenges they present.
Prepare to expand your geometric horizons and gain an unparalleled understanding of the circular world that surrounds us.
Circle Definitions and Properties
A circle is a plane figure that consists of all points in a plane that are equidistant from a given point, called the center. The distance from any point on the circle to the center is called the radius.
Circles are found in many real-world applications, such as wheels, gears, and pulleys. They are also used in architecture, engineering, and design.
The properties of circles include:
- The radius is half the diameter.
- The circumference is the distance around the circle.
- The area of a circle is given by the formula A = πr^2.
Measuring Circles

The formulas for calculating the radius, diameter, and circumference of a circle are:
- Radius (r) = Diameter (d) / 2
- Diameter (d) = 2 – Radius (r)
- Circumference (C) = π – Diameter (d)
These formulas can be used to solve problems involving circles. For example, if you know the radius of a circle, you can use the formula for circumference to find the distance around the circle.
Circle Relationships
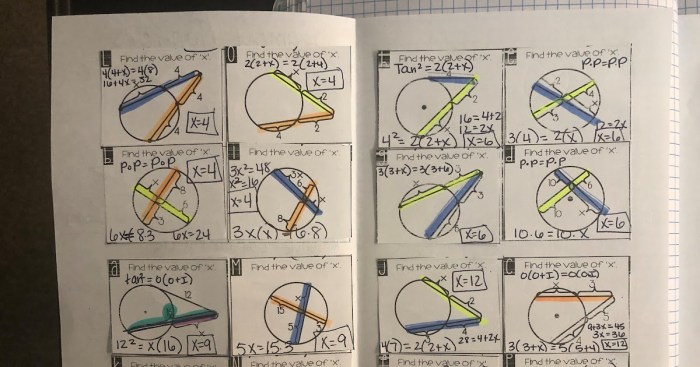
The radius, diameter, and circumference of a circle are related by the following formulas:
- Circumference (C) = 2π – Radius (r)
- Diameter (d) = Circumference (C) / π
- Radius (r) = Circumference (C) / 2π
These relationships can be used to solve problems. For example, if you know the circumference of a circle, you can use the formula for radius to find the radius of the circle.
Circle Geometry Applications
Circles are used in a variety of fields, such as:
- Architecture
- Engineering
- Design
For example, circles are used to design wheels, gears, and pulleys. They are also used in the construction of buildings and bridges.
Additional Circle Concepts: Common Core Geometry Unit 9 Circle Geometry Answers
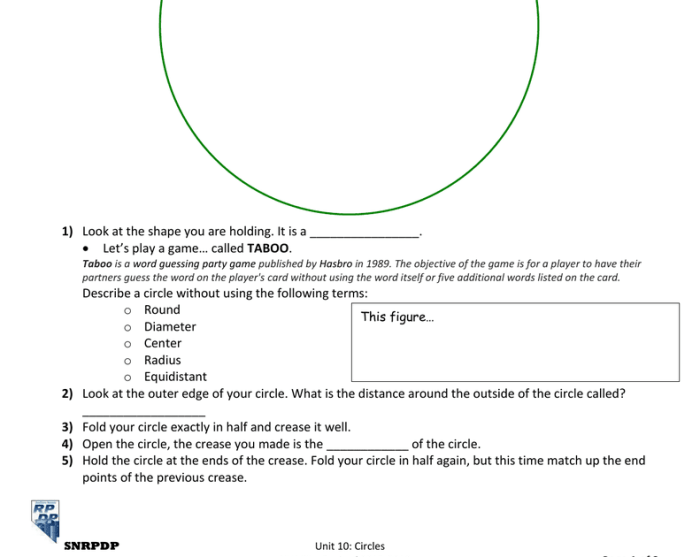
In addition to the basic properties of circles, there are a number of other concepts that are related to circles, including:
- Inscribed circles
- Circumscribed circles
- Tangent circles
These concepts are used in a variety of applications, such as geometry, engineering, and design.
Questions and Answers
What is the formula for the circumference of a circle?
C = 2πr, where C is the circumference, π is a constant approximately equal to 3.14, and r is the radius of the circle.
How do I find the area of a circle?
A = πr², where A is the area and r is the radius of the circle.
What is the relationship between the radius, diameter, and circumference of a circle?
The circumference of a circle is equal to π times the diameter, or C = πd, where d is the diameter.